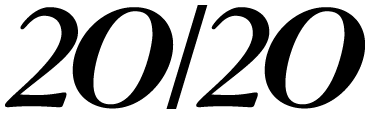By Brent McCardle

One of the first formulas we learn in ophthalmic optics is about prism. Naturally that involves learning Prentice’s Rule, Δ=c×F. The formula is quite simple. Prism is the difference in centimeters from the optical center to another point, multiplied by the power of the lens in diopters.

When you inspect a plano lens in the lensmeter, you will notice some slight movement in the mires. Yet according to Prentice’s Rule, this should not be the case. Why the discrepancy?

I was able to find the answer in a paper written by Don Whitney, an American Optical lens designer. The paper was titled, “Prentice’s Rule—Its Applications and Limitations.” In his paper, Whitney shows how Prentice’s Rule will break down in lower power lenses. But before we can understand why it breaks down, we need to understand how Prentice’s Rule was derived.

Figure 1 shows a prism where light is refracted which creates an angle of deviation(d) from its original path. The amount it is deviated from its original path is indicated by x over a distance of F. To solve for the angle of deviation we use: (1) tan(d)=x/F .

One prism diopter is indicated quite simply as a ratio of 1 in 100. We can use any unit, but usually we say a ray is deviated 1 cm over a distance of 1 m.

By knowing this, we can rearrange this formula to state (2) Δ=100[tan(d)], where Δ is prism.

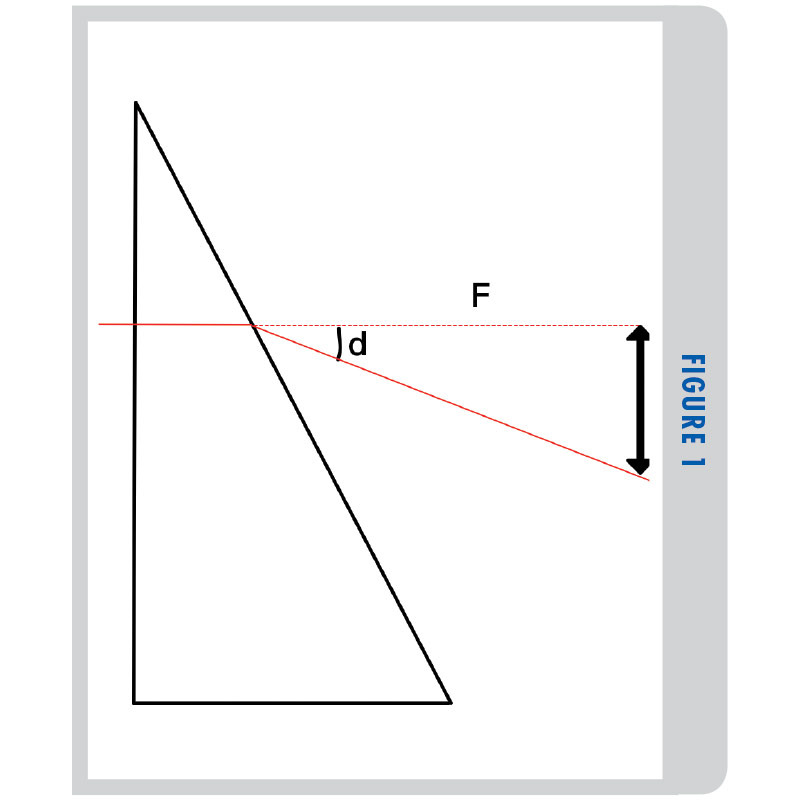
Figure 2 shows a minus lens where one surface is plano, and the other surface is curved. With the prism from Figure 1, you can see that a ray of light strikes the front surface and is refracted from the back surface. The focal length of the lens is indicated by F, and the dioptric power is the reciprocal of the focal length:

D=1/F. Now we can combine the first two formulas to say that Δ=(100)x/(1/D), which then can be reduced to Δ=(100)(x)(D), which is Prentice’s Rule.

We can see from the derivation, that Prentice’s Rule requires that one surface be plano while the other surface will produce the required refraction.

Most lower powered lenses use two curved surfaces, while higher powers feature one surface flatter. It is for this reason that Prentice’s Rule will become less reliable in lower powered lenses.

If we were to calculate Prentice’s Rule using a lens of plano power, it will calculate to 0 diopters of prism, regardless of how much distance we move from the optical center. However, when we place a plano lens in the lensmeter, and we move the lens, we notice that there is a slight amount of prism. This is due to the fact that a lens of plano power is not made using the same curvature on the front and the back surfaces.

If you consider the front and back surfaces as elements in an optical system separated by the thickness, we find that anytime we separate two refracting surfaces, it will introduce positive power. Let’s say a plano lens with a 2.2 mm center thickness and a refractive index of 1.586 on a 6.00 D base curve. Since base curves are usually marked in 1.53 index, we should convert the surface to the true refracting power by multiplying the base curve by the tooling index (usually 1.53) - 1 and divide this by the index of the lens minus 1.

D1=(6×0.53)/0.586=6.63 D, this is known as the refractive front power. We can then determine the back power needed for a plano lens by: D₂=D-(D₁/(1-(t_m/n)D₁), where D is plano in this case, tm is the thickness in meters and n is the index of refraction. After calculating we can see that D2 is -6.70 D. With D1 equal to 6.63 and D2 equal to -6.70, we see that we need to create a steeper curve on the back side to create more minus power. This will offset for the plus power created by the thickness and curvature from the front surface. Because the two curves are not equal, we see a slight amount of prism as we move the lens in the lensmeter.

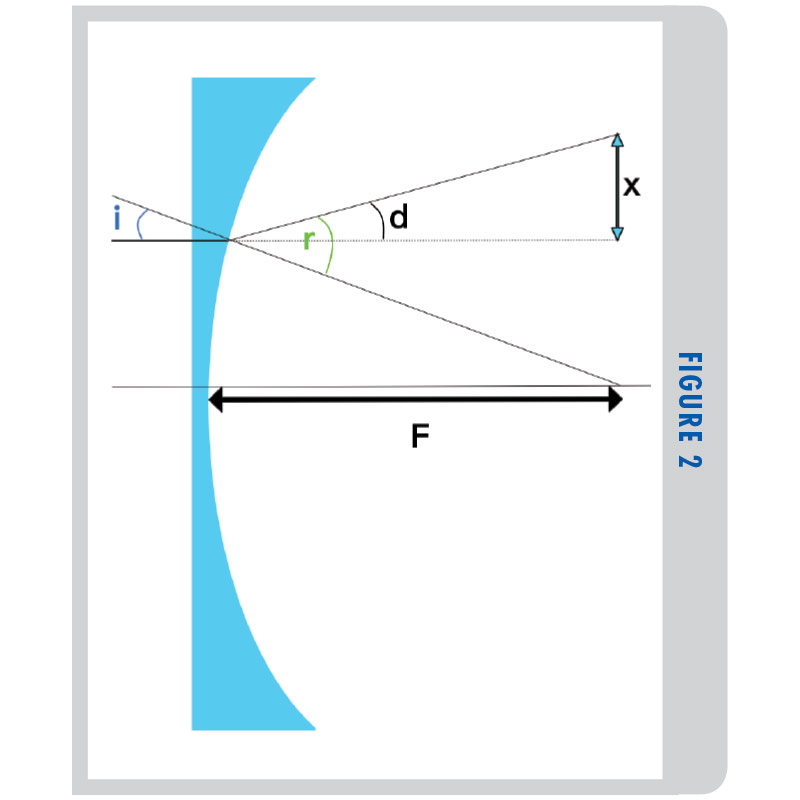
The only lens that would not create prism when moved away from the optical center is one whose radii of curvature differ only by the thickness of the lens which would be a concentric lens as seen in Figure 3. We see that the separation between R1 and R2 is the 2.2 mm thickness of the lens.

We can now determine the true power of each surface by D₁=(n-1)/r_m and D₂=(1-n)/r_m, where the index is equal to 1.586, D1 = 6.63 D and D2 = -6.80 D

Since most lenses will not meet the criteria of a concentric lens, how do we calculate prism for lower powers if Prentice’s Rule is not as accurate? We will need to ray trace the back surface of the lens.

From Figure 4 you can see that the ray the back surface radius creates an angle with the optical axis(w), which intersects the lens at a height of x. We can determine the angle of incidence by first finding angle w:
w=sin-¹(x/r2)

Now we can find the distance from r1 to r2 on the optical axis, labeled z:
z=r₂-r₁+t

To find the angle of incidence, we use the law of sines: z/sini=r₁/sinw, and rearrange to solve for i: sini=zsinw/r₁

Now that we have the angle of incidence, we can find the angle of refraction from Snell’s Law: (n_r)(sinr)=(n_i)(sini), we can rearrange to solve for r: r=sin^-1 [(n_i)(sini)].

Since we have the angle of incidence and the angle of refraction, we can now find the angle of deviation, and from that we can find the prism amount.

d=i-r
Δ=100(tand)

Whitney and his colleague, John Winthrop, were able to come up with a more digestible formula than what is listed above, but more precise than Prentice’s Rule.

Δ=100(P1+P2-[t/(n-1)][P1+P2])x, where:
P1 is the true front power
P2 is the true back power
t is the thickness in meters
n is the refractive index

Using the above formula, we will plot the difference between Don Whitney’s calculation and Prentice’s Rule. The range of power will be from +1.50 to -1.50. Each lens will have a 6.00 D base curve with a 2.2 mm center, and we will use 1.586 material. We are going to calculate each power 5 mm from its optical center or x = 5.

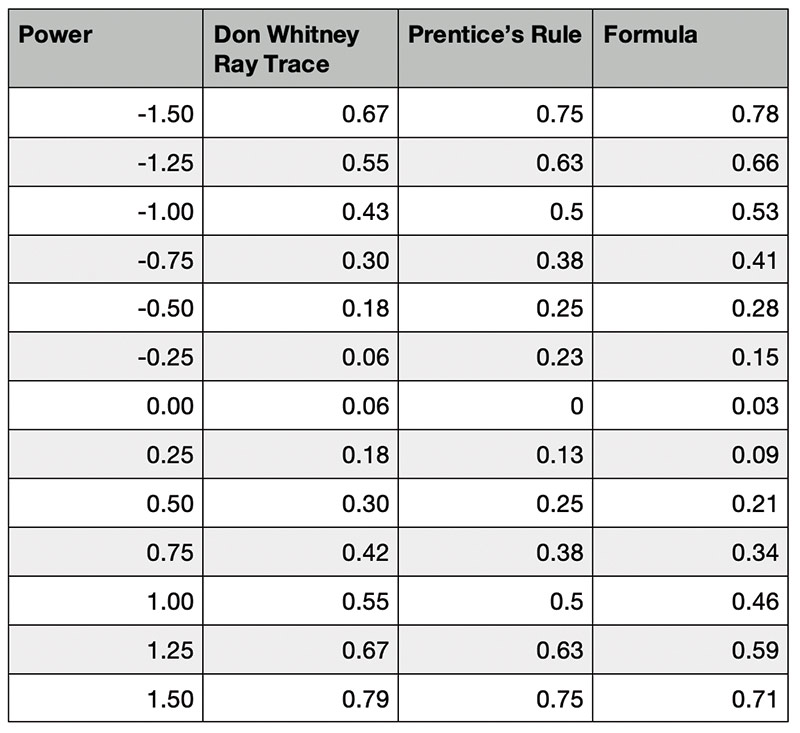
This exercise is probably more academic than most would like to see, however it shows that the more we understand how a lens is constructed and where some of our more basic formulas are derived, it will help us to better understand patient complaints. Don Whitney’s article opened up the world of ophthalmic optics for me and started me on the path of always wanting to understand everything I can.

---

Brent McCardle is a licensed optician with over 35 years of experience. His role as technical education specialist at Zeiss provides him the opportunity to work on new product innovations with marketing and to work closely with the training of eyecare professionals.