Base curves, we've learned are an effective way to provide adequate vision, over the entire lens, for a range of sphere and cylinder prescriptions. However, as lens power departs from the ideal power for that base curve, vision gets progressively more blurred in the periphery i.e., the clear field of view shrinks.

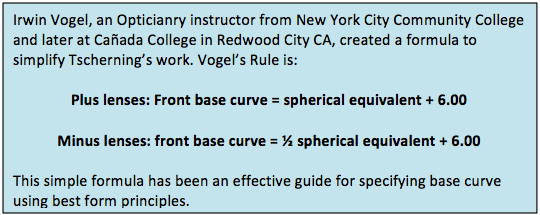
I taught Opticianry for many years with Professor Irwin Vogel and he created a simple and effective formula for opticians for specifying base curves. This formula considered the management of off axis astigmatism that creates blur. (For a detailed explanation of off axis errors, see the Best Form Lens Design section of the CE course Ophthalmic Lens Design by Darryl Meister.)

However, best form optics base curves don't work as well for digital lenses because they are often flatter than those originally specified. Arbitrary base curves ordered by opticians could create other problems; if too flat for plus prescriptions, adds could be biconvex and if too steep, require additional calculations. So, a clever optician, Brian Boddy from Acoma Optical in Santa Fe developed this new formula for specifying base curves when ordering digital lenses (in fact it has become his submission in a paper for his ABO Masters).

Brian writes:
Being one of the first retail stores to surface and brand private label progressives, we enjoyed a freedom to sell almost any lens material in any lens option using backside progressive or free-form technology. Unfortunately many times we outpaced our LMS (Laboratory Management System) system due to the sheer volume of lenses and digital progressives that required updating.

On one occasion a dispenser sold a progressive in violet polarized plastic that needed to be done in a day! With little demand for such a unique almost one of a kind lens it became necessary to create a formula for my lab personnel to follow when filling these truly unique and very custom backside digital progressive lens orders.

THE BODDY FORMULA

For minus digital lenses:
4.25 + ((Spherical equivalent + add)/2)

I replaced Vogel's Rule of thumb ocular curve from the 6.00 diopters to 4.25 because the 4.25 diopter number more accurately represents the aspheric mean of a plano lens when placed on a 4.25 diopter base curve. Digital lenses are designed aspheric for spherical Rx's and atoric for cylindrical Rx's on the backside of the lens.

For plus digital lenses is:
3.50 + ((add power/2) + Spherical equivalent)

In plus prescriptions, use 3.50 diopters as the rule for two reasons. The first is addressed above; the lens will be aspheric and/or atoric on the backside. The second reason is, unlike Vogel's Rule incorporating the add power into the base curve equation assuring that the resulting base curve choice will not be too flat or become bi-convex so the result is the best optically performing lens.

Let's look at the prescription above and apply the Boddy Formula to it:

OD +2.25 sphere, 3.00 add
OS +2.25 sphere, 3.00 add

The Boddy Formula calculation would be:

3.50 + ((add power/2) + Spherical equivalent)
= 3.50 + ((3.00/2) + 2.25)
= 3.50 + (3.75)
= 7.25 D

Applying my formula suggests using a 7.25 D base curve. While this a diopter less than Vogel's Rule suggestion of 8.25 diopter base curve it is above the 6.00 diopter base curve most laboratories would grind this prescription on. Why would I suggest a higher base curve?

Here is why my formula works. At the NRP the resulting ocular curve would be -2.00, which is an optimal aspheric backside curve. If we used a 6.00 diopter base curve lens the resulting curve at NRP would be -1.00, below the manufacturer recommended threshold for quality aspheric lenses.

Have a comment? Let us know.